The Moon and Eclipses
After the sun, the most noticeable celestial object is the moon. Its motions, phases, and
occasional eclipses add delightful variety to our sky. It's also the closest
astronomical object by far.
The Moon's Motions
The moon's motion through our sky is similar to that of the sun, but different:
- Like the sun, the moon rises in the east and sets in the west (with some exceptions for observers in
earth's extreme northern and southern regions).
- Like the sun, the moon doesn't move across our sky quite as fast as the stars do.
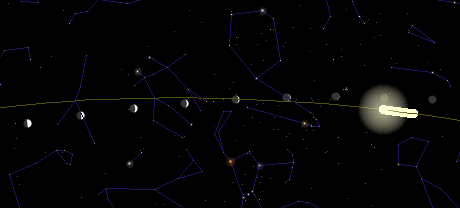
- However, whereas the sun creeps eastward only one degree per day with respect to the stars,
the moon moves eastward, with respect to the stars, by about 13 degrees per day (see illustration
below). This means that if you pay attention, you can easily notice the moon's motion, with
respect to the stars, during a single night.
- As a consequence, the moon moves eastward with respect to the sun by about 12 degrees
per day (13 minus 1). This means that the moon rises later and later each successive day, sometimes rising
in the morning, sometimes in the afternoon, and sometimes at night. Similarly, the moon sets later
each day, and can
set at any time of the day or night, depending on where it is with respect to the sun.
- At a rate of 12 degrees per day, the moon completes a full circle, with respect to the sun,
about once every 30 days (actually 29.5). This time period was originally called one month
(guess where the word comes from), although our modern calendar is not directly tied to
the moon and defines different months to have different lengths.
- The moon is always seen near the ecliptic, but its motion carries it about five degrees
to either side during the course of each month.
Question: Suppose, for the purpose of this question, that a month
is exactly 30 days. How many minutes later, on each successive day, would you expect the
moon to rise, on average? Remember that after a full month (30 days), the moon should rise
at the same time as originally (neglecting seasonal variations).
The Moon's Phases
Besides moving around the sky, the moon is famous for its phases: its variety
of apparent shapes, from crescent to half to gibbous to full. There is also a "new" phase,
when we don't see the moon at all for a few days. What causes these striking changes
in the moon's appearance?
Here are some big clues:
- First, the moon goes through its complete cycle of phases exactly once
each (lunar) month, that is, in the same time period as its motion with respect to the sun.
- Second, the
moon's phase is always new when it is near
the sun in our sky, and
full when it is opposite the sun,
with intermediate phases at intermediate angles (between the moon and sun).
- Third, when the moon is in its crescent phase and the sky is sufficiently dark, you can
sometimes make out the rest of the moon's
dimly lit disk. This tells us that the moon isn't
really changing shape; part of it is merely much brighter than the rest.
- Finally, at any phase between new and full, you can check that the illuminated side of
the moon is always the side that's facing
toward the sun.
The simple explanation of all these observations is that the moon shines by reflected sunlight.
It's spherical in shape, with half of the sphere illuminated by the sun at any given time.
How much of this half we see, however, depends on our viewing direction. When the moon and sun
are near each other in our sky, we're looking at the moon's dark side so we don't see it at
all ("new moon"). When the moon is opposite the sun in our sky, we're looking at the moon's
illuminated side so we see a full moon. At other angles we see part, but not all, of the
moon's illuminated side. The illustration below shows how you can simulate the moon's phases using
a ball and a strong directional light source to simulate the sun.
The moon's new and crescent phases occur when the sun is more or less behind the moon, from
our perspective. Just looking at these phases, therefore, tells us immediately that the
moon must be closer to us than the sun. There's even a way to estimate how much closer it is,
as I'll explain below.
Question: Assuming you live at a mid-northern latitude, where would you look
in the sky to see a crescent moon at sunset?
Question: At what time of day or night does the moon rise when it's full?
Question: Many scenic photographs of the moon are faked, by combining
two separate photos.
Think of some ways you can tell when the moon has been artificially added to a photo.
Solar Eclipses
By an amazing coincidence, the moon's apparent size in our sky is almost
exactly the same as the sun's: about half a degree. This means that it's possible, as the new moon
passes by the sun in its monthly trip around the ecliptic, for the moon's disc to completely
cover the sun. When it does, our
sky becomes dark for a few minutes
and we can see the
thin, hot
gases surrounding the sun, called the solar corona. This
dramatic event is called a total
solar eclipse.
Remember, though, that the moon can wander as much as five degrees to either side of the ecliptic.
In most months, therefore, the new moon misses the sun (from our perspective) by a few degrees,
to one side or the
other, as it passes. But the moon's path crosses the ecliptic roughly twice each month, and a solar eclipse
occurs when the sun happens to be there during the crossing. There's a good chance of this about
once every six months. Even then, however, perfect alignment requires that you be
somewhere along
a narrow path across earth's surface, usually less than 300 km wide.
And even then, the moon's disc isn't always quite
big enough to cover the sun, because both of their apparent sizes vary as their distances from
earth vary slightly. When the moon is too far and/or the sun is too close, yet the alignment is
good, we call the event an
annular eclipse.
Otherwise, if the moon covers only part of the sun,
we call it a partial solar eclipse.
Whether the eclipse is total (or annular) or not, a partial solar eclipse is usually visible
over a wide area about twice each year. I've seen several partial solar eclipses over the years,
and if you're old
enough, you probably have as well. I've never been in the right place to see a total solar eclipse,
but I'm looking forward to the one that will come through much of the U.S. on
August 21, 2017.
Lunar Eclipses
An equally interesting, if less dramatic, event occurs when the sun and
moon are exactly
opposite each other in our sky. This is when the moon is normally full, and very bright. But if the
alignment is precise enough, the earth will block the sun's light from reaching the moon. This event
is called a lunar eclipse.
As with solar eclipses, there's a good chance for a lunar eclipse to
occur about once every six months. Some are only partial, with the earth's shadow never covering
more than
part of the moon, because the alignment is imperfect.
Your chances of having seen a total
lunar eclipse are pretty good, because they're visible from everywhere on the night side of the
earth, and the totality can last over an hour. Clouds can get in the way, of course, and
you sometimes need to be willing to get up in the middle of the night.
The next lunar eclipse as of this writing will be a total one on
December 10, 2011; best viewed
from the Asia-Pacific region, it will also be visible in the morning, as the moon is setting,
from western North America. For the next two years the
lunar eclipses
will be only partial, with the
next total lunar eclipse not occurring until
April 15, 2014.
Although you might expect the moon to become totally dark during a lunar eclipse, in fact a little
sunlight still gets to it—by bending around the earth, through earth's atmosphere. The same
thing happens to us every evening after the sun sets, when we still see light in the western sky.
And just as with sunsets, it's the red component of the sunlight that best penetrates earth's
atmosphere and reaches the eclipsed moon.
The Moon's Size
Besides just being a cool sight to watch, a lunar eclipse gives us a way to determine
the moon's true size.
As shown at right, the curvature of earth's shadow on the partially eclipsed moon tells us
the size of earth's shadow, in comparison to the moon. Specifically, the diameter of earth's shadow
is a little less than three times the diameter of the moon. In other words, the moon's diameter
is a little more than one third that of the shadow.
If earth's shadow were the same size as the earth itself, we could immediately conclude that the
moon's diameter is a little over a third that of the earth. But it's not that simple, because
earth's shadow tapers off, getting narrower as you get farther and farther away (as shown in the
lunar eclipse diagram above). To take this tapering into account, you have to do a rather
intricate calculation
using several principles of geometry and algebra. The bottom line is that
the earth's shadow, at the moon's location, is about 3/4 as wide as the earth itself. Therefore,
since the moon is a little over 1/3 as wide as the shadow, it must be a little over 1/4 as wide
as the earth. In miles, the earth's diameter is about 8000 so the moon's diameter is about 2000.
This ingenious method of determining the moon's size was devised by the Greek
astronomer Aristarchus of Samos,
in the third century B.C. Although the method is absolutely
sound, Aristarchus used rather inaccurate measurements so his numerical answer for the moon's size
wasn't especially good. But later Greek astronomers repeated the calculation, using better measurements.
Even without doing any calculations, however, you can just look at a partial lunar eclipse and see
that the moon is probably just a few times smaller than the earth. Of course, you can also see
that earth's shadow is curved. Aristotle, the great Greek philosopher, used this observation to argue
that the earth must be a sphere.
The Distance to the Moon
Knowing the moon's true diameter (1/4 that of the earth), we can use its
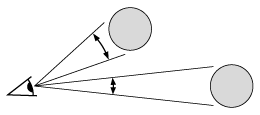
measured angular diameter (half a degree) to determine its distance. Qualitatively, the idea
is this: For a given true size, a larger angular diameter would mean that the moon must be closer;
whereas a smaller angular diameter would mean that the moon must be farther away. Because we
know the moon's angular diameter, we can calculate just how far it is.
The calculation takes a few steps, and requires some effort and practice
to fully understand. But similar calculations come up so often in astronomy that it's a good
investment to start practicing now.
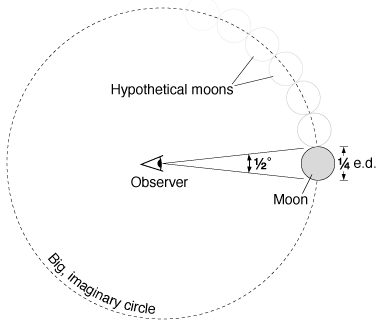
A useful trick for visualizing the calculation is to sketch a big, imaginary circle, centered on you
(the observer), passing through the moon. Then label the angle that represents the moon's measured
angular width (half a degree in reality, but it's fine to exaggerate it in the picture as I have).
Also label the moon's known diameter, 1/4 (in units of earth's diameter).
The calculation proceeds in three steps:
- Ask yourself how many hypothetical moons it would take, placed side by side, to reach all the way
around the circle. Since a full circle is 360° but each moon takes up only 1/2°, the
answer is 360 divided by 1/2, or 720 hypothetical moons.
- Now calculate the circumference of the circle. Since each moon has a width of 1/4 e.d., and
it takes 720 of them to go all the way around, the answer is 720 times 1/4 e.d., or 180 earth
diameters. (You could use units of miles instead of earth diameters if you prefer, but then
the numbers get a little more cumbersome: 720 × 2000 = 1440000.)
- The distance to the moon is the radius of the circle. But for any circle, the
circumference is 2π times the radius, where π is a number that's equal to a little more
than 3. If you don't need an especially accurate answer, it's fine to pretend that π equals 3
and just say that the circumference is six times the radius. Then the radius (or distance) is
simply the circumference divided by 6, or in this case, 180 e.d. divided by 6, or 30 earth diameters.
I like to call these kinds of calculations big circle problems. The general idea
is that if you know the angular size and the true size of an object, you can calculate its
distance by drawing a big circle and going through these three steps. A slight variation, which
comes up just as often, is when you already know the distance and the angle, and want to calculate
the true size. Please make sure you understand each step of the calculation, and practice until
you can put them together and do a whole big circle problem on your own.
Question: Imagine that you live on an alien world with a moon that
appears only half as wide as ours: 1/4 degree. You also know that the true diameter of
this moon is 500 miles. Sketch a big circle, centered on you and passing through the moon,
and label it with the angle and true diameter. How many hypothetical moons would fit around the
full circle?
Next step: What is the circumference of the circle, in miles?
Final step: Using the approximation π = 3, what is your estimate of the
distance to this moon, in miles?
The next two questions are harder, so you may want to skip them the first time your read this.
Be sure to come back and work them, for practice, when you have time!
Question: Bruno the Bully is chasing you across the playground.
Being the nerdy type, you decide to stop running away and figure out just how far from you
he is (so you can then calculate how long you have to live).
You know that Bruno is 6 feet tall, and, holding up your outstretched fist,
you estimate his angular height to be five degrees. Using the approximation π = 3,
what is your estimate of Bruno's distance in feet?
Question: According to your map, the mountain on the horizon is
30 kilometers away. Holding out your fist, you estimate its angular height to be three degrees.
How tall is the mountain, in kilometers? (Again, please use the approximation π = 3.)
Coming back to the moon, our calculation gave a distance of 30 earth diameters (or 60 earth
radii, or about a quarter million miles). Here is a picture of the earth and moon, to scale:
Comprehending this enormous distance is a challenge even today, when automobile odometer mileages
often exceed a quarter million. But imagine how astonishing this distance must have been
to the ancient Greeks, who traveled only by the power of muscle and wind. Equally astonishing
is that the Greeks could determine this distance without the benefit of
space travel or even a telescope. As we'll see over and over again, the human intellect can reach
out to measure things that are far beyond our physical reach.
The Distance to the Sun
Besides calculating the moon's distance, Aristarchus also devised an equally
ingenious method to estimate the distance to the sun.
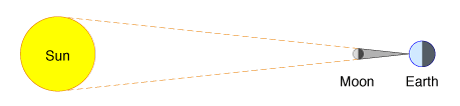
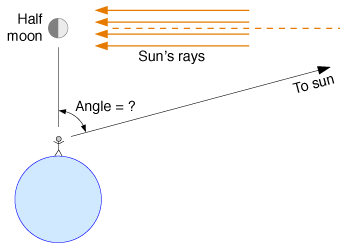
Wait until the moon is exactly half full, then look up at it. If the illuminated half of the
moon's sphere is on your right, this means that the sunlight must be coming directly from
the right, at a 90-degree angle to your line of sight. So on the diagram, you know
that the sun must lie somewhere along the dashed orange line.
To determine where along the dashed orange line, all you have to do is measure the angle
between the moon and the sun, from your perspective. Point to the moon with one hand and the sun with
the other, and estimate the angle between your arms. Then, on the diagram, measure out the same
angle and extend a line from
you toward the sun. Draw the sun where that line intersects the dashed orange line.
If you actually try this procedure, though, you'll find that the angle is indistinguishable from 90°.
It has to be slightly less, of course, but you can't tell how much less because it's
impossible to tell when the moon is exactly half full. But you can see, at least, that
since the angle is close to 90°, the sun must be many times farther away than the moon.
Aristarchus wrote that the angle between the half-moon and the sun was 87°, and from
this, estimated that the sun is about 19 times as far away as the moon. What he probably should
have said is that the sun is at least 19 times as far away. And since the sun and moon
have about the same angular size, this means that the sun's true diameter is at least 19 times that
of the moon. Thus, Aristarchus was able to prove that the sun must be much
bigger than the earth—a result that must have been hard for anyone to believe at the time.
We now know that the sun is almost 400 times as far away as the moon—an even more astonishing
result. Because this distance is so large, nobody was able to measure it until after the invention
of the telescope, 1900 years after the time of Aristarchus. But even without a telescope,
you can use the method of Aristarchus to see that the sun must be many times farther away
than the moon. Next time you see a half-full moon, look at where the sun is and then try to visualize
them in three dimensions!
Question: Suppose you see a half-full moon in the southern sky, about
half-way between the horizon and zenith. As you look toward it, the illuminated half is
on the right. What time of day or night is it?
Copyright ©2010-2011 Daniel V. Schroeder.
Some rights reserved.