The Ring Nebula. Image credit: Howard Bond, STScI
Summary

The student will examine many of the properties of planetary nebulae, including their composition, size and evolution.

Background and Theory

A planetary nebula is formed when a red giant star approaches the end of its life span and begins to lose a lot of mass very quickly. This mass condenses, and forms a shell around the star. This cloud of dust and gas obscures the central star for a time. The star moves to the left across the H-R diagram. This means that the temparature radically increases, while the luminosity remains approximately constant. The hot star begins emitting ultraviolet light, which ionizes the gas in the nebula. This ionized gas begins to glow, making the planetary nebula luminous. Eventually, the central star becomes fully evolved, and its luminosity falls by as much as 90%. The cool star is no longer capable of ionizing the nebula, so the nebula gradually fades and disperses into the interstellar medium. Planetary nebulae are responsible for a large fraction of the mass returned to the interstellar medium each year.

Planetary nebulae emit visible light of many different colors, depending upon which atoms are present. Blue-green nebulae contain OIII (oxygen ions), while red nebulae are dominated by hydrogen and nitrogen.

Procedure

Print out the worksheet.

Part A: Chemical Composition
  1. Each of the images on the screen is of a different planetary nebula. Examine each image. Make a table of your own that lists the nebulae and a short description of what you see in the image. Comment on the shape of the nebula, and whether it looks the same in all colors.
  2. These nebulae shine in the visible wavelengths because of line emission. Do the Hydrogen and Oxygen emission come from the same place in the nebula? In general, which comes from closer in, and which further out? Are there any exceptions?
  3. Think back to the explanation of how lines are formed. Electrons in hydrogen are loosely bound, compared to the electrons in this particular oxygen transition. Does the overall trend in the location of the colors make sense? Explain.

Part B: Changing Mass Loss Rate

  1. Examine the image of the Ring Nebula at the top of the page. The brighter regions of the image are places where the density of the nebula is high, since there is more material to create emission. Material close to the central star was probably lost recently, while material far from the star was probably lost some time ago. Make a plot of the mass loss versus time by beginning at the central star, and moving toward the lower right corner, plotting the brightness of the nebula on the y-axis, and time on the x-axis. Label your axes with arrows indicating increasing brightness, and increasing age.

  2. One way to interpret these data are to guess that the nebula is actually a hollow sphere. Then the middle is dim because there is less material along the line of sight, while all around the outside, the nebula is bright where you are looking through the material at an angle. This model is shown at right. 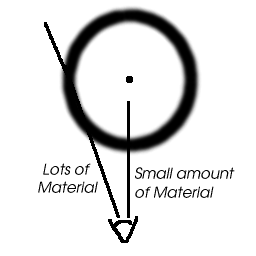Diagram of limb-brightening of a hollow sphere. How do your observations differ from this model? How are they consistent?

  3. Has this mass loss been constant over time? Describe the history of the mass loss from the central star.

Part C: Size and Age of the Dumbbell Nebula
  1. Measure the long axis of the Dumbbell Nebula (in pixels). (Need the distance formula?)

  2. Divide this by 2 to get the radius in pixels.

  3. The image scale for this image is 3 arcseconds/pixel. Multiply the radius of the Dumbbell nebula by this image scale to get the angular radius, A of the nebula.

  4. Now, use the small angle formula to find the actual radius, R of the nebula in kilometers. The distance, D, to M27 is 3.4X1016 km (3,500 light years), and the small angle formula is:

    R=D*A/206,265

  5. We can assume that the expansion speed of the nebula is about 20 km/s. Find the age of the Dumbbell Nebula. Don't forget to convert the age from seconds to years so that you have a sensible answer!

Part D: Mass Return Rate
  1. Use the formula for the volume of a sphere: V=(4/3)R3 to find the volume of the Dumbbell Nebula.

  2. The density of the nebula is very low, n=1.7X10-10kg/km3. Multiply this density by the volume to get the total mass in the Dumbbell Nebula in kilograms.

  3. The entire mass of this nebula will become a part of the interstellar medium. There are about 700 planetary nebulae in our galaxy. Estimate the mass returned to the interstellar medium each year by multiplying the mass of the Dumbbell by the number of nebulae, and dividing by the lifetime of the Dumbbell (in years), as found in Part C. Convert this mass return rate to solar masses by dividing by the mass of the Sun (Msun=2X1030 kg). Is this a large amount of material?

© 2007 Weber State University
Revised: 10/2007