Planet Discovery Worksheet

  1. A period is defined as one complete cycle; that is, where the radial velocities return to the same position on the curve (but at a later time).
    How many cycles did the star go through during the 14 days of observations?
    Number of cycles = ___________

  2. What is the period, P, in days?
    Period = ___________ days

  3. What is P in years?
    P = _____________ years

  4. What is the uncertainty in your determination of the period? That is, by how many days or fractions of a day could your value be wrong?
    Uncertainty = ___________ days
  5. What is the amplitude, K? (Take 1/2 of the value of the full range of the velocities.)
    K = ___________ m/s

  6. How accurate is your determination of this value?
    Uncertainty = __________ m/s


  7. We will make some simplifying assumptions for this new planetary system:

    1. the orbit of the planet is circular (e = 0)
    2. the mass of the star is 1 solar mass
    3. the mass of the planet is much, much less that of the star
    4. we are viewing the system nearly edge on
    5. we express everything in terms of the mass and period of Jupiter

    We make these assumptions to simplify the equations we have to use for determining the mass of the planet. The equation we must use is:

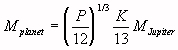
    determining mass of a planet

    P should be expressed in years (or fraction of a year), and K in m/s. Twelve years is the approximate orbital period for Jupiter and 13 m/s is the magnitude of the "wobble" of the Sun due to Jupiter's gravitational pull. Not all calculators will take the cube root of a number. Get help if yours does not. Put in your values for P and K and calculate the mass of this new planet in terms of the mass of Jupiter. That is, your calculations will give the mass of the planet as some factor times the mass of Jupiter (for example: Mplanet = 4 MJupiter). Show all work.









  8. Assume that the parent star is 1 solar mass, and that the planet is much less massive than the star. We can then calculate the distance this planet is away from its star, in astronomical units (AU's) by using Kepler's third law:
    Kepler's 3rd Law

    Again, P is expressed in years (or fraction of a year), and a represents the semi-major axis in AU's. Solve for a:
    Distance in AU's

    a = __________ AU

  9. Compare this planet to those in our solar system. For example, Mercury is 0.4 AU from the Sun; Venus, 0.7 AU; Earth, 1.0 AU; Mars, 1.5 AU; Jupiter, 5.2 AU. Jupiter is more massive than all the rest of the matter in the solar system combined, excluding the Sun.



  10. What is unusual about this new planet?



  11. Science is based upon the ability to predict outcomes. However, nothing prepared astronomers for the characteristics of this "new" solar system. Why was it such a surprise?



  12. If this actually is a planet, is it possibly hospitable to life? Explain.





  13. Name your new planet -- a privilege you would have if you really did discover a new planet!