Curvature of Space

Circle Limit IV: Heaven and Hell by M.C. Escher (1960)

Purpose
In this lab we'll have a look at curvature and some examples from other "universes". Then we'll examine how we might apply that knowledge to our own.
Procedure
Print the worksheet.

Imagine yourself in "Flatland", a 2-dimensional flat space, like an infinite piece of paper. If a sphere passed down through the plane of Flatland, you would first see a point, which would grow to a circle, reach a maximum size, shrink to a point again and disappear. Suppose a 3-dimensional cube passes through Flatland. What shapes could you see?
What might a 4-dimensional sphere look like if it passed it through 3-dimensional space?
It's very hard to imagine a 3-dimensional space that's curved into a fourth dimension, so we have to refer to 2-dimensional spaces that are curved into a third dimension as an analogy. Imagine that you are a 2-dimensional creature living on the surface of a sphere. List three geometrical tests that would tell you if your universe is positively curved.
The Dutch graphic artist M.C. Escher is known for his surreal and illusional works. In many of these works, he used the concept of tiling (the regular division of a plane into tiles) to investigate the question of curvature. The two works in this assignment are projections of creatures in 2-dimensional spaces, which may or may not be flat, onto the page, which is definitely flat. The creatures are all the same size in their own world. The apparent change in size of the angels and devils in Circle Limit V is an artifact of the projection onto the flat page, like the distortion of the size of Greenland on a Mercator map in an atlas. We want to determine the curvature of the original space.
Geometry can help us with this. First of all, on any surface, of any curvature, the sum of the angles at any point is equal to 360 degrees. Secondly, the sum of the interior angles of a triangle will be exactly 180 degrees for a flat surface, less than 180 degrees for a negatively curved surface, and more than 180 degrees for a positively curved one.
In these works, the creatures are tiled, so that their bodies fit together in regular patterns. To find the curvature, we can find a repeating triangular shape and count how many triangles intersect at different points. From this you can determine the size of each of the angles of the triangle.

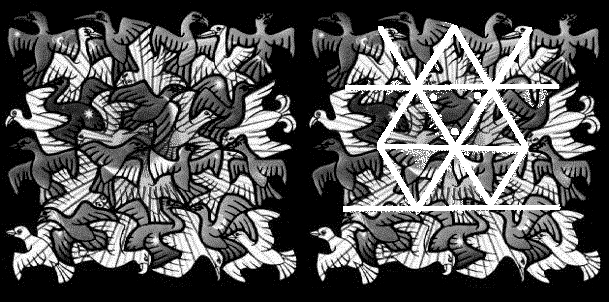
Figure 2: Sun and Moon by M.C.Escher (1948).
In Figure 2, there are two types of birds, which are all roughly triangular (their vertices being the points where 6 birds are touching: the wing-tips and the beaks). The birds are all the same size in their space, so that the 6 angles at a vertex are all the same. There are always six birds coming together at a vertex, so that each of the interior angles (the white dots on the image to the right) of each bird's triangle is:

360 degrees / 6 = 60 degrees. The sum of the three angles of the triangle of each bird is therefore (60 + 60 + 60 =) 180 degrees, so that we now know that the curvature is flat.
First, guess the curvature represented in Figure 3.
Figure 3: Circle Limit 4 -- (Heaven and Hell) by M.C.Escher (1960).
Now, use the method from the example to find the curvature by counting triangular tiles meeting at a vertex point. Note that in this case the triangles are not equilateral. Show your work.
Enough with those 2-dimensional analogies. Let's move on to our real universe and see how geometry might tell us things interesting to astronomers and you. How might you use two beams of light to figure out what the local curvature of our universe is? The local curvature isn't necessarily the same as the global curvature at all. What dominates the curvature in the inner solar system? Is it flat, positive or negative? How do we know?
Could we geometric methods to determine the curvature of the entire universe? Why or why not?